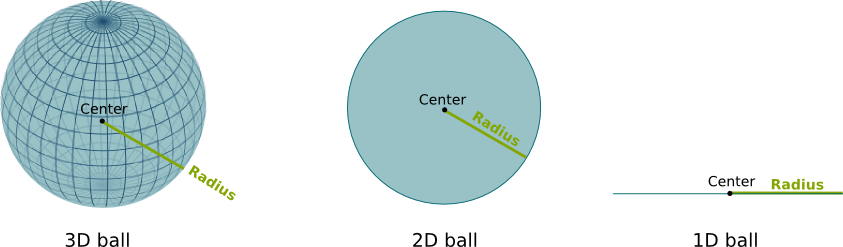
Tag Archives: Topology
The Poincaré conjecture | Relativité 21
Can you glue opposite edges of a square? Relativity 7
Topology, Homotopy and Poincaré’s Conjecture (Trek through Math 3/8)
Graphs and the 4 Color Theorem (Trek through Math 2/8)
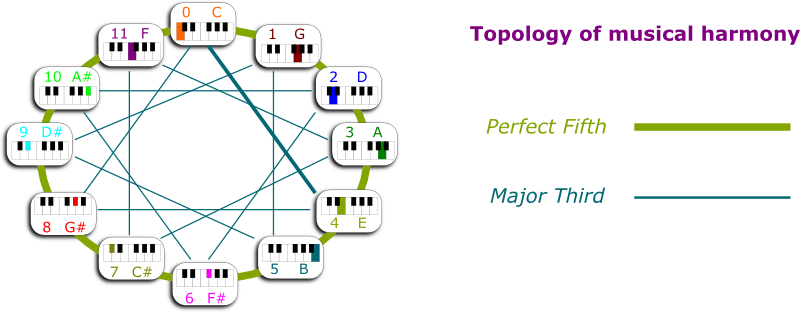
The Harmonious Mathematics of Music
The Magic of Analysis

The Addictive Mathematics of the 2048 Tile Game

Univalent Foundations of Mathematics
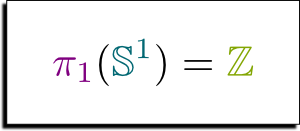
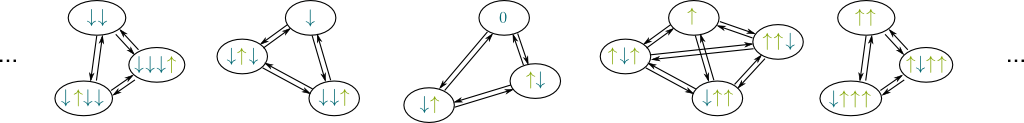
Homotopy Type Theory and Higher Inductive Types
The Tortuous Geometry of the Flat Torus
Imaginary and Complex Numbers
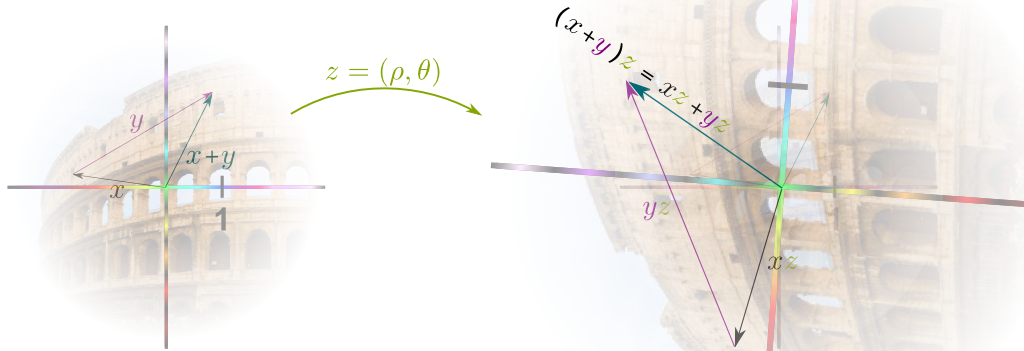
Euler’s Formula and the Utilities Problem
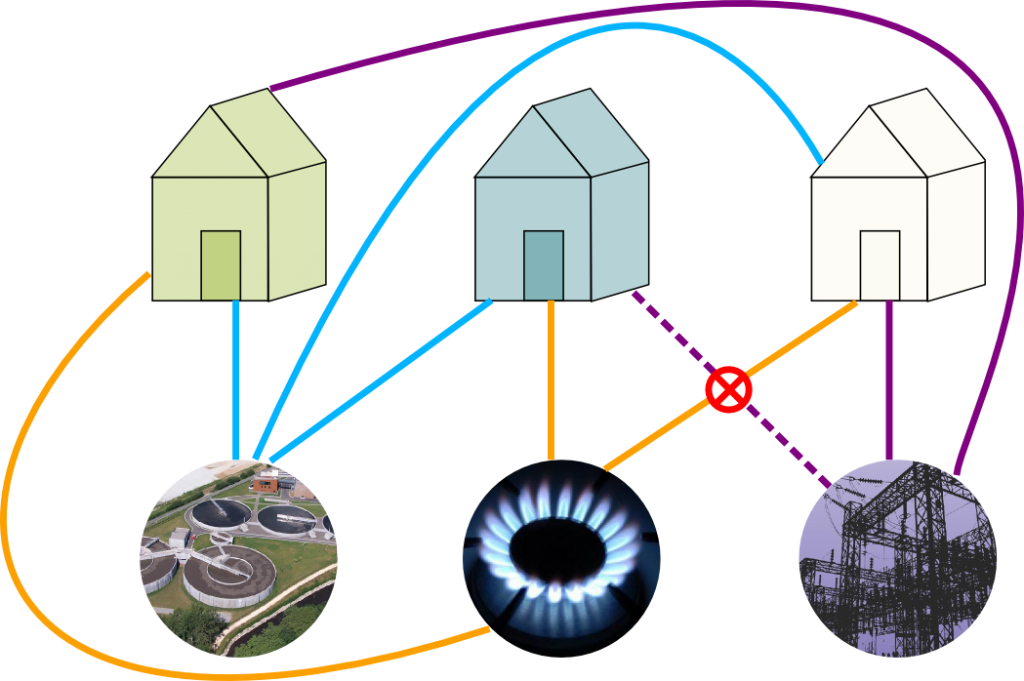
Poincaré Conjecture and Homotopy
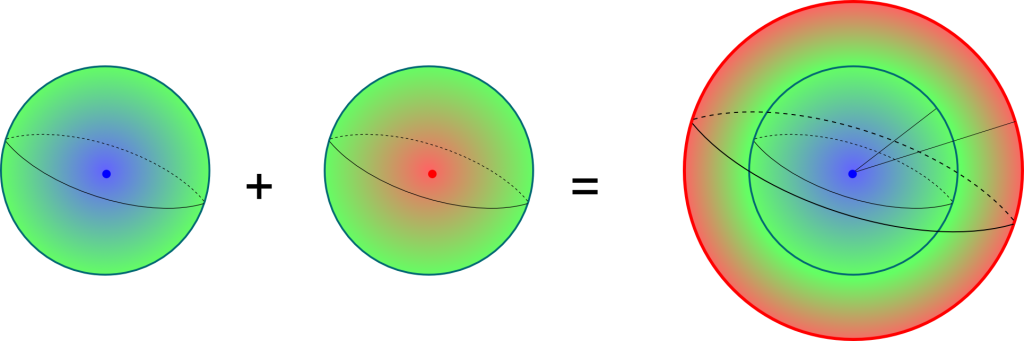
Topology: from the Basics to Connectedness